Gen Math Lesson 2 i1e2o

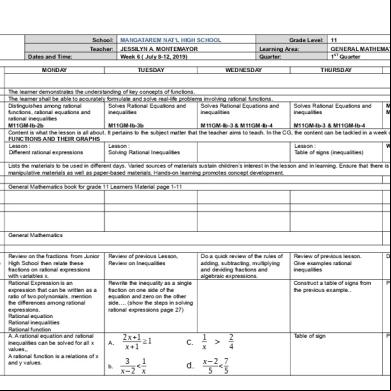
LESSON PLAN IN GENERAL MATHEMATICS I. LEARNING OBJECTIVES At the end of the lesson, the students are able to:  performs addition, subtraction, multiplication, division and composition of functions ; and  solves problems involving functions. II. LEARNING CONTENT A. Subject Matter: Functions and Their Graphs B. Lesson:

Operations on Functions ( Lesson 3)

C. Code:

M11GM-Ia-3 and M11GM-Ia-4

D. References:

General Mathematics by Orlando A. Oronce , pp. 10- 15

E. Materials:

Manila paper, pentel pen

F. Strategies:

Cooperative Learning

III. LEARNING ACTIVITIES/PROCEDURES A. Preliminary Activity  Prayer  Checking of Attendance  Drill : Integer Flashcard Activity B. Review  How do we evaluate functions? C. Lesson Proper 1. Motivation Present a scenario where the different operations can be performed on functions solicited from the students. 2. Presentation Students are divided in groups where they will be given problems to g ( x)  x  1 answer and discuss in class: f ( x)  x 2  2 a. Solve for f ( x)  g ( x) b. Solve for f ( x)  g ( x) c. Solve for f ( x)  g ( x) f ( x) d. Solve for g ( x) e. Solve for f ( x)  g ( x)

3. Discussion Highlight the idea to the students that in performing addition, subtraction, multiplication, division and composition of functions they must consider previously learned concepts on integers and algebra. 4. Developmental Activity The students will be given word problems involving function. Example #1:

The function for the height of an object dropped from a 100-meter tall platform at time t seconds is approximated by s(t )  5t 2  100 . What is the height of the object after 2 seconds? After 4 seconds? 5. Synthesis/Generalization  What are the important techniques to use in solving problems involving functions?

IV. EVALUATION 1. Perform the indicated operations: Given: f ( x)  2 x 2 g ( x)  x  2 a. Solve for f ( x)  g ( x) b. Solve for f ( x)  g ( x) c. Solve for f ( x)  g ( x) f ( x) d. Solve for g ( x) e. Solve for f ( x)  g ( x) 2. Solve the problem. Show complete solution. Mark started selling snacks in the nearby school. In one day, he spends P200.00 for rent and P25.00 for each snack item he prepares. His expenses in a single day can be expressed as a function C ( x)  25 x  200 , where x is the number of items and C(x) is his daily expenses in pesos. How much are his expenses if he prepares 100 snack items? 150 snack items?

V. ENRICHMENT A problem set involving operations of functions will be given to students.