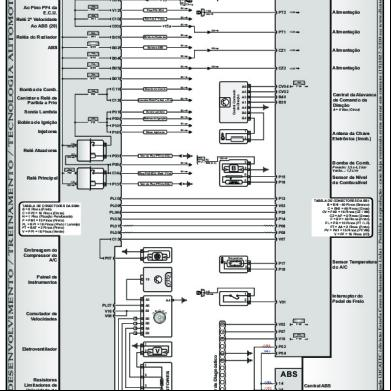
C3 Trigonometry 2o1i6n
This document was ed by and they confirmed that they have the permission to share it. If you are author or own the copyright of this book, please report to us by using this report form. Report 2z6p3t
Overview 5o1f4z
& View C3 Trigonometry as PDF for free.
More details 6z3438
- Words: 2,280
- Pages: 13
C3 Trigonometry
-1-
Secant, Cosecant, Cotangent 1.
Sec x =
1 , cos x
cos x ≠ 0
2.
Cosec x =
1 , sin x
sin x ≠ 0
3.
Cot x =
1 , tan x
tan x ≠ 0
=
cos x , sin x
sin x ≠ 0
On your calculator, you will have to use sin, cos and tan keys, followed by the 1 −1 reciprocal key or x . However, to find exact values for the 'special' x angles, follow the following examples. Examples Find the exact value of: 1
(i) (ii) (iii) (iv)
2.
(i) (ii) (iii)
sec 60 o cosec 60 o cot 30 o cosec 45 o π cosec 6 π sec 4 π cot 3
Solution With calculaor in degrees 1. (i) (ii)
1 Press cos 60 because sec = to get 0.5 then press 1/x to get 2 cos press sin 60 to get 018660 ... which isn't a recognisable fraction ... then press x2 to get 0.75 ( = 34 ) i.e. sin2 60 =
3 4
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 1
C3 Trigonometry
-2-
3 2
so, sin 60 =
2
so, cosec 60 = (iii)
3
press tan 30 to get 05773 ... which isn’t a recognisable fraction ... then press to get 0.3333 ... which is 31 . x2 i.e. tan2 30 = so, tan 30 =
1 3 1 3
so, cot 30 o =
(iv)
3
press sin 45 o to get 0.7071 ... which is not a recognisable fraction ... then press x2 to get 0.5 ( = 21 ) i.e. sin2 45 =
1 2 1
so, sin 45 =
2
so, cosec 45 =
2
with calculator in rads: 2. (i)
press sin ( π ÷ 6) to get 0.5 ( =
1 2
)
∴ cosec
π =2 6
(ii) press cos ( π ÷ 4) to get 0.7071 ... then press i.e.
cos2
so, cos
sec,
π = 4
π = 4
π = 4
x2
1 2
1 2
2
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 2
to get 0.5 ( =
1 2
).
C3 Trigonometry (iii)
-3-
Press tan ( π ÷ 3 ) to get 1.732 ... then press i.e. tan2
x2
π =3 3
tan
π = 3
cot
1 π = 3 3
3
The graphs of sec, cosec and cot are shown below:
y = cosecθ
θ
y = secθ θ
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 3
to get 3
C3 Trigonometry
-4-
y = cot θ
Their period are the same as cos, sin and tan. Also,
Sin+
All+
Tan+
Cos+
⇒
Cosec+
All+
Cot+
Sec+
Trigonometric Identities You already know sin2 θ + Cos2 θ ≡ 1 If you divide by Cos θ 2
2 1 → sin 2θ +1 ≡ cos2 θ cos θ
We get
tan2 θ + 1 ≡ sec2 θ
similarly, dividing by sin2 θ gives
1 + cot2 θ ≡ cos ec 2θ
These can be used to simplify expressions
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 4
C3 Trigonometry
-5-
Example (i)
Express 5cot θ + 2 cosec2 θ in of cot θ
(ii)
Solve the equation 5 cot θ + 2 cosec2 θ = 5 for 0 < θ < 2 π
Solution (i)
5 cot θ + 2 cosec2 ≡ 5 cot θ + 2 (1 + cot2 θ ) ≡ 5 cot θ + 2 + 2cot2 θ
(ii)
5 cot θ + 2 cosec2 θ = 5 ⇒ 5 cot θ + 2 + 2 cot2 θ = 5 ⇒ 2 cot2 θ + 5 cot θ - 3 = 0
This is a quadratic in cot θ cot θ =
=
−5 ± 25 − 4(2)( −3) 2x2 −5 ± 49 −5 ± 7 1 = = 2 or -3 4 4
⇒ tan θ = 2 or - 31
+ve tan ∴ 1st and 3rd quad.
-ve tan ∴ 2nd and 4th quad
Because the range of θ is given in of π we must have our Calculator in radians
θ = tan −1 2 = 1.11
θ = 1.11, π + 1.11 =1.11, 4.25
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 5
C3 Trigonometry
-6-
1 θ = tan −1 − = −0.322 3
The Addition Formulae For all angles, A and B, sin ( A ± B ) = sin A cos B mcos A sin B
cos ( A ± B ) = cos A cos B msin A sin B tan ( A ± B ) =
tan A ± tan B 1 mtan A tan B
(these are in the formula book) o o Example 1: Prove the identity sin ( x + 45 ) + cos ( x + 45 ) = 2 cos x
Solution:
sin ( x + 45o ) = sin x cos 45 + cos x sin 45 1 1 + cos x x 2 2 cos ( x + 45) = cos x cos 45 − sin x sin 45 = sin x x
1 1 − sin x x 2 2
= cos x x
cos x cos x + 2 2 1 1 = cos x + 2 2 2 = cos x x 2 = 2 cos x
∴ sin ( x + 45 ) + cos ( x + 45) =
Example 2: By writing 75o as (30o + 45o) find the exact value of cos75o
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 6
C3 Trigonometry
-7-
Solution:
cos75 = cos(30 + 45) = cos30cos45 - sin30sin45 3 2 1 2 = x − x 2 2 2 2 6 2 = − 4 4 Example 3: Find the value of tanxo given that sin(x + 30o) = 2cos(x – 30o) Solution:
sin(x + 30) = 2cos(x - 30) sin x cos 30 + cos x sin 30 = 2[cos x cos 30 + sin x sin 30] 3 1 3 1 sin x + cos x = 2 [ cos x + sin x] 2 2 2 2 = 3 cos x + sin x
Rearranging gives 3 1 sin x − sin x = 3 cos x − cos x 2 2 3 1 sin x = 1 = cos x 3 − 2 2 3 1 tan x − 1 = 3 − 2 2 1 3− 2 tan x = 3 −1 2 2 3 −1 = 3−2
( ÷ cos x )
2 × 2
Double Angle Formulae(LEARN) Putting A=B into the addition formulae gives identities for sin2A, cos2A and tan2A:Using,
sin(A+B) = sin A cos B + cos A sin B.
Sin 2A = sin(A+A) = sin A cos A + cos A sin Sin 2A = 2 sin A cos A Similarly
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 7
C3 Trigonometry
-8-
cos 2 A = cos2 A − sin2 A = 2cos2 A − 1 = 1 − 2 sin2 A Note: there are 3 forms of cos2A and tan 2A =
2 tan A 1 − tan2 A
Example: Solve the equation 2 sin 2θ = sin θ for 0 ≤ θ ≤ 360o (to 1dp) Solution: 2 sin 2θ
= sinθ
4 sinθ cos θ
= sinθ
2 [ 2 sinθ cos θ ] = sinθ ( Do not divide by sin θ. You must factorise)
4 sin θ cos θ − sin θ = 0 sin θ (4 cos θ − 1) = 0
sinθ = 0
or
θ = 0,180o,360o
4 cos θ − 1 = 0 cos θ = 0.25
θ = 75.5o,284.5o
θ = 0o,75.5o,180o,284.5o,360o
Example;
If sin A =
3 and A is obtuse, find the exact values of cos A, 5
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 8
C3 Trigonometry
-9-
sin2 A, tan 2 A. Solution: sin2 A + cos2 A = 1 ⇒ cos2 A = 1 − sin2 A 9 16 = 1− = 25 25 4 ∴ cos A = ± , but A is obtuse. 5 4 ∴ cos A = − 5 sin2 A = 2 sin A cos A 3 4 = 2 × × (− ) 5 5 24 =− 25 sin A 3 2 tan A tan A = = − ⇒ tan2 A = cos A 4 1 − tan2 A 2( − 34 ) − 32 24 = = 7 =− 9 1 − 16 7 16
Solve cos 2A + 3 + 4cos A = 0, 0 ≤ A ≤ 2π
Example: Solution:
(Since we have cos A in the equation we choose the form of cos 2A which involves cos A only) cos 2 A = 2cos2 A − 1
i.e.
i
Sub in equation : (2cos2 A − 1) + 3 + 4cos A = 0 2cos2 A + 4 cos A + 2 = 0 cos2 A + 2cos A + 1 = 0 (this is a quadratic in cosA) −2 ± 4 − 4 2 = −1
cos A =
A = cos -1 ( −1) A=π The form a cos x + b sin x ≡ R cos( x ± ∝), R sin( x ± ∝ )
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 9
C3 Trigonometry
- 10 -
It is useful to be able to express the sum of sin x and cos x as a single sin or cos. It enables us to: - find the max/min of the expression - solve equations To find R and ∝ , we expand the right hand side and equate like . This gives R = + a 2 + b2 ← ( ALWAYS ) ∝ ∝ and values for sin and cos in of a, b and R. Example: i) Express 3sinx+2cos x in the form Rsin( x + ∝) ii) Find the max and min of the expression and find in radians to 2dp the smallest positive value of x for which they occur. iii) Solve the equation 3 sin x + 2 cos x = 1 Solution: (i)
for -π ≤ x ≤ π to 2dp
R= 32 + 22 = 13
3 sin x + 2cos x ≡ R sin( x + ∝) 3 sin x + 2cos x ≡ R sin x cos ∝ +R cos x sin ∝ 3 sin x + 2cos x ≡ (R cos ∝)sin x + (R sin ∝)cos x Equating sin x :→ Equating cos x :→
3 = R cos ∝ ....(1) 2 = R sin ∝ ....(2)
(2) ÷ (1) gives tan ∝=
2 3
2 ∝= tan−1( ) = 0.5880 and R= 13, from above 3 ∴ 3 sin x + 2cos x = 13 sin( x + 0.5880) ii) Max and min of 3 sin x + 2 cos x is same as max & min of 13sin(x+0.5880) Max of any sine is +1 Min of any sine is -1 ∴ Max of expression is + 13 and Min of expression is - 13 Max when sin (x +0.5880) = 1 ∴ x + 0.5880 = π , x = 0.9828 2 = 0.98c (2dp) Min when sin ( x + 0.5880) = −1 ∴ x + 0.5880 = 3π x = 4.12c (2dp ) 2 /opt/idoub/conversion/tmp/scratch25351/31244900.doc 10
C3 Trigonometry
iii )
- 11 -
3 sin x + 2cos x = 1 13 sin( x + 0.5880) = 1 ⊥ 13 1 x + 0.5880 = sin−1( ) 3 x + 0.5880 = 0.2810. sin( x + 0.5880) =
x + 0.5880 = 0.2810, = 0.2810, x = −0.307, = −0.31, NB:
i.e
π − 0.2810 2.8606 2.7226 2.72 (2dp )
3sin x + 2cos x can also be expressed in the form R cos ( x + ∝ ), but it is usually more convenient to choose the form which produces the in the right order with the correct sign. a sin x - b cos x = R sin ( x − ∝) a cos x + b sin x = R cos ( x − ∝) a cos x - b sin x = R cos ( x + ∝)
Inverse Trig Functions For f -1(x) to exist then f ( x ) must be a one to one function. If f(x) Is not 1-to-1 over its whole domain then f −1( x ) can exist provided we restrict the domain of f (x) The functions cos x,sin x and tan x are not 1-to-1 over their whole domain. The inverse functions cos-1 x, sin-1 x and tan−1 x exist for the domains:
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 11
C3 Trigonometry
- 12 0≤ x ≤π −π ≤ x ≤ π 2 2 −π π <x< 124 2 4 32
cos−1 x : sin−1 x : tan−1 x :
(tan x has asymptotes at ±
π ) 2
The graph of f −1 (x) is a reflection of f(x) in the line y = x ∴ sin−1,cos−1 x,tan−1 x are reflections of sin x, cos x tan x in the line y = x (x in radians, same scale on both axes).
π 2
π 4
−
π 2
-1
f ( x ) = sin −1 x
1 −
π 4
−
π 2
π 2
f ( x ) = arcsin x π
π 2
−
π 2
-1
1
π 2
f ( x ) = cos −1 x
f ( x ) = arccos x
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 12
C3 Trigonometry
- 13 -
π 2
−
π 2
-1
1
−
π 2
π 2
f ( x ) = tan −1 x
f ( x ) = arctan x
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 13
-1-
Secant, Cosecant, Cotangent 1.
Sec x =
1 , cos x
cos x ≠ 0
2.
Cosec x =
1 , sin x
sin x ≠ 0
3.
Cot x =
1 , tan x
tan x ≠ 0
=
cos x , sin x
sin x ≠ 0
On your calculator, you will have to use sin, cos and tan keys, followed by the 1 −1 reciprocal key or x . However, to find exact values for the 'special' x angles, follow the following examples. Examples Find the exact value of: 1
(i) (ii) (iii) (iv)
2.
(i) (ii) (iii)
sec 60 o cosec 60 o cot 30 o cosec 45 o π cosec 6 π sec 4 π cot 3
Solution With calculaor in degrees 1. (i) (ii)
1 Press cos 60 because sec = to get 0.5 then press 1/x to get 2 cos press sin 60 to get 018660 ... which isn't a recognisable fraction ... then press x2 to get 0.75 ( = 34 ) i.e. sin2 60 =
3 4
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 1
C3 Trigonometry
-2-
3 2
so, sin 60 =
2
so, cosec 60 = (iii)
3
press tan 30 to get 05773 ... which isn’t a recognisable fraction ... then press to get 0.3333 ... which is 31 . x2 i.e. tan2 30 = so, tan 30 =
1 3 1 3
so, cot 30 o =
(iv)
3
press sin 45 o to get 0.7071 ... which is not a recognisable fraction ... then press x2 to get 0.5 ( = 21 ) i.e. sin2 45 =
1 2 1
so, sin 45 =
2
so, cosec 45 =
2
with calculator in rads: 2. (i)
press sin ( π ÷ 6) to get 0.5 ( =
1 2
)
∴ cosec
π =2 6
(ii) press cos ( π ÷ 4) to get 0.7071 ... then press i.e.
cos2
so, cos
sec,
π = 4
π = 4
π = 4
x2
1 2
1 2
2
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 2
to get 0.5 ( =
1 2
).
C3 Trigonometry (iii)
-3-
Press tan ( π ÷ 3 ) to get 1.732 ... then press i.e. tan2
x2
π =3 3
tan
π = 3
cot
1 π = 3 3
3
The graphs of sec, cosec and cot are shown below:
y = cosecθ
θ
y = secθ θ
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 3
to get 3
C3 Trigonometry
-4-
y = cot θ
Their period are the same as cos, sin and tan. Also,
Sin+
All+
Tan+
Cos+
⇒
Cosec+
All+
Cot+
Sec+
Trigonometric Identities You already know sin2 θ + Cos2 θ ≡ 1 If you divide by Cos θ 2
2 1 → sin 2θ +1 ≡ cos2 θ cos θ
We get
tan2 θ + 1 ≡ sec2 θ
similarly, dividing by sin2 θ gives
1 + cot2 θ ≡ cos ec 2θ
These can be used to simplify expressions
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 4
C3 Trigonometry
-5-
Example (i)
Express 5cot θ + 2 cosec2 θ in of cot θ
(ii)
Solve the equation 5 cot θ + 2 cosec2 θ = 5 for 0 < θ < 2 π
Solution (i)
5 cot θ + 2 cosec2 ≡ 5 cot θ + 2 (1 + cot2 θ ) ≡ 5 cot θ + 2 + 2cot2 θ
(ii)
5 cot θ + 2 cosec2 θ = 5 ⇒ 5 cot θ + 2 + 2 cot2 θ = 5 ⇒ 2 cot2 θ + 5 cot θ - 3 = 0
This is a quadratic in cot θ cot θ =
=
−5 ± 25 − 4(2)( −3) 2x2 −5 ± 49 −5 ± 7 1 = = 2 or -3 4 4
⇒ tan θ = 2 or - 31
+ve tan ∴ 1st and 3rd quad.
-ve tan ∴ 2nd and 4th quad
Because the range of θ is given in of π we must have our Calculator in radians
θ = tan −1 2 = 1.11
θ = 1.11, π + 1.11 =1.11, 4.25
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 5
C3 Trigonometry
-6-
1 θ = tan −1 − = −0.322 3
The Addition Formulae For all angles, A and B, sin ( A ± B ) = sin A cos B mcos A sin B
cos ( A ± B ) = cos A cos B msin A sin B tan ( A ± B ) =
tan A ± tan B 1 mtan A tan B
(these are in the formula book) o o Example 1: Prove the identity sin ( x + 45 ) + cos ( x + 45 ) = 2 cos x
Solution:
sin ( x + 45o ) = sin x cos 45 + cos x sin 45 1 1 + cos x x 2 2 cos ( x + 45) = cos x cos 45 − sin x sin 45 = sin x x
1 1 − sin x x 2 2
= cos x x
cos x cos x + 2 2 1 1 = cos x + 2 2 2 = cos x x 2 = 2 cos x
∴ sin ( x + 45 ) + cos ( x + 45) =
Example 2: By writing 75o as (30o + 45o) find the exact value of cos75o
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 6
C3 Trigonometry
-7-
Solution:
cos75 = cos(30 + 45) = cos30cos45 - sin30sin45 3 2 1 2 = x − x 2 2 2 2 6 2 = − 4 4 Example 3: Find the value of tanxo given that sin(x + 30o) = 2cos(x – 30o) Solution:
sin(x + 30) = 2cos(x - 30) sin x cos 30 + cos x sin 30 = 2[cos x cos 30 + sin x sin 30] 3 1 3 1 sin x + cos x = 2 [ cos x + sin x] 2 2 2 2 = 3 cos x + sin x
Rearranging gives 3 1 sin x − sin x = 3 cos x − cos x 2 2 3 1 sin x = 1 = cos x 3 − 2 2 3 1 tan x − 1 = 3 − 2 2 1 3− 2 tan x = 3 −1 2 2 3 −1 = 3−2
( ÷ cos x )
2 × 2
Double Angle Formulae(LEARN) Putting A=B into the addition formulae gives identities for sin2A, cos2A and tan2A:Using,
sin(A+B) = sin A cos B + cos A sin B.
Sin 2A = sin(A+A) = sin A cos A + cos A sin Sin 2A = 2 sin A cos A Similarly
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 7
C3 Trigonometry
-8-
cos 2 A = cos2 A − sin2 A = 2cos2 A − 1 = 1 − 2 sin2 A Note: there are 3 forms of cos2A and tan 2A =
2 tan A 1 − tan2 A
Example: Solve the equation 2 sin 2θ = sin θ for 0 ≤ θ ≤ 360o (to 1dp) Solution: 2 sin 2θ
= sinθ
4 sinθ cos θ
= sinθ
2 [ 2 sinθ cos θ ] = sinθ ( Do not divide by sin θ. You must factorise)
4 sin θ cos θ − sin θ = 0 sin θ (4 cos θ − 1) = 0
sinθ = 0
or
θ = 0,180o,360o
4 cos θ − 1 = 0 cos θ = 0.25
θ = 75.5o,284.5o
θ = 0o,75.5o,180o,284.5o,360o
Example;
If sin A =
3 and A is obtuse, find the exact values of cos A, 5
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 8
C3 Trigonometry
-9-
sin2 A, tan 2 A. Solution: sin2 A + cos2 A = 1 ⇒ cos2 A = 1 − sin2 A 9 16 = 1− = 25 25 4 ∴ cos A = ± , but A is obtuse. 5 4 ∴ cos A = − 5 sin2 A = 2 sin A cos A 3 4 = 2 × × (− ) 5 5 24 =− 25 sin A 3 2 tan A tan A = = − ⇒ tan2 A = cos A 4 1 − tan2 A 2( − 34 ) − 32 24 = = 7 =− 9 1 − 16 7 16
Solve cos 2A + 3 + 4cos A = 0, 0 ≤ A ≤ 2π
Example: Solution:
(Since we have cos A in the equation we choose the form of cos 2A which involves cos A only) cos 2 A = 2cos2 A − 1
i.e.
i
Sub in equation : (2cos2 A − 1) + 3 + 4cos A = 0 2cos2 A + 4 cos A + 2 = 0 cos2 A + 2cos A + 1 = 0 (this is a quadratic in cosA) −2 ± 4 − 4 2 = −1
cos A =
A = cos -1 ( −1) A=π The form a cos x + b sin x ≡ R cos( x ± ∝), R sin( x ± ∝ )
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 9
C3 Trigonometry
- 10 -
It is useful to be able to express the sum of sin x and cos x as a single sin or cos. It enables us to: - find the max/min of the expression - solve equations To find R and ∝ , we expand the right hand side and equate like . This gives R = + a 2 + b2 ← ( ALWAYS ) ∝ ∝ and values for sin and cos in of a, b and R. Example: i) Express 3sinx+2cos x in the form Rsin( x + ∝) ii) Find the max and min of the expression and find in radians to 2dp the smallest positive value of x for which they occur. iii) Solve the equation 3 sin x + 2 cos x = 1 Solution: (i)
for -π ≤ x ≤ π to 2dp
R= 32 + 22 = 13
3 sin x + 2cos x ≡ R sin( x + ∝) 3 sin x + 2cos x ≡ R sin x cos ∝ +R cos x sin ∝ 3 sin x + 2cos x ≡ (R cos ∝)sin x + (R sin ∝)cos x Equating sin x :→ Equating cos x :→
3 = R cos ∝ ....(1) 2 = R sin ∝ ....(2)
(2) ÷ (1) gives tan ∝=
2 3
2 ∝= tan−1( ) = 0.5880 and R= 13, from above 3 ∴ 3 sin x + 2cos x = 13 sin( x + 0.5880) ii) Max and min of 3 sin x + 2 cos x is same as max & min of 13sin(x+0.5880) Max of any sine is +1 Min of any sine is -1 ∴ Max of expression is + 13 and Min of expression is - 13 Max when sin (x +0.5880) = 1 ∴ x + 0.5880 = π , x = 0.9828 2 = 0.98c (2dp) Min when sin ( x + 0.5880) = −1 ∴ x + 0.5880 = 3π x = 4.12c (2dp ) 2 /opt/idoub/conversion/tmp/scratch25351/31244900.doc 10
C3 Trigonometry
iii )
- 11 -
3 sin x + 2cos x = 1 13 sin( x + 0.5880) = 1 ⊥ 13 1 x + 0.5880 = sin−1( ) 3 x + 0.5880 = 0.2810. sin( x + 0.5880) =
x + 0.5880 = 0.2810, = 0.2810, x = −0.307, = −0.31, NB:
i.e
π − 0.2810 2.8606 2.7226 2.72 (2dp )
3sin x + 2cos x can also be expressed in the form R cos ( x + ∝ ), but it is usually more convenient to choose the form which produces the in the right order with the correct sign. a sin x - b cos x = R sin ( x − ∝) a cos x + b sin x = R cos ( x − ∝) a cos x - b sin x = R cos ( x + ∝)
Inverse Trig Functions For f -1(x) to exist then f ( x ) must be a one to one function. If f(x) Is not 1-to-1 over its whole domain then f −1( x ) can exist provided we restrict the domain of f (x) The functions cos x,sin x and tan x are not 1-to-1 over their whole domain. The inverse functions cos-1 x, sin-1 x and tan−1 x exist for the domains:
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 11
C3 Trigonometry
- 12 0≤ x ≤π −π ≤ x ≤ π 2 2 −π π <x< 124 2 4 32
cos−1 x : sin−1 x : tan−1 x :
(tan x has asymptotes at ±
π ) 2
The graph of f −1 (x) is a reflection of f(x) in the line y = x ∴ sin−1,cos−1 x,tan−1 x are reflections of sin x, cos x tan x in the line y = x (x in radians, same scale on both axes).
π 2
π 4
−
π 2
-1
f ( x ) = sin −1 x
1 −
π 4
−
π 2
π 2
f ( x ) = arcsin x π
π 2
−
π 2
-1
1
π 2
f ( x ) = cos −1 x
f ( x ) = arccos x
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 12
C3 Trigonometry
- 13 -
π 2
−
π 2
-1
1
−
π 2
π 2
f ( x ) = tan −1 x
f ( x ) = arctan x
/opt/idoub/conversion/tmp/scratch25351/31244900.doc 13